How to Convert Effective Rate to Continuous Rate
Formula to Calculate Effective Annual Rate (EAR)
The formula of Effective Annual Rate Effective annual rate (EAR) is the rate actually earned on investment or paid on the loan after compounding over a given period of time and is used to compare financial products with different compounding periods i.e. weekly, monthly, annually, etc. As the compounding periods are increased, the EAR increases. Effective Annual Rate = (1 + i/n)n – 1 read more (EAR) can be calculated based on the nominal rate of interest Nominal Interest rate refers to the interest rate without the adjustment of inflation. It is a short term interest rate which is used by the central banks to issue loans. read more and number of compounding periods per year.
The effective annual rate is also known as an effective rate, or an annual equivalent rate, is the rate of interest that is actually earned or pay after compounding, and it is calculated by one plus annual interest rate, which is divided by a number of compounding periods to the power number of periods whole minus one.
Effective Annual Rate = (1 + r/n)n – 1
where r = Nominal interest rate of interest and n = number of compounding periods per year.

You are free to use this image on your website, templates, etc, Please provide us with an attribution link Article Link to be Hyperlinked
For eg:
Source: Effective Annual Rate Formula (wallstreetmojo.com)
However, in the case of continuous compounding formula The continuous compounding formula depicts the interest received when constant compounding is done for an infinite number of periods. The four variables used for its computation are the principal amount, time, interest rate and the number of the compounding period. read more , the equation of effective annual rate is modified as below,
Effective Annual Rate = er – 1
The effective annual rate is also known as an effective interest rate Effective Interest Rate, also called Annual Equivalent Rate, is the actual rate of interest that a person pays or earns on a financial instrument by considering the compounding interest over a given period. read more , annual equivalent rate, or effective rate.
Table of contents
- Formula to Calculate Effective Annual Rate (EAR)
- Steps to Calculate Effective Annual Rate (EAR)
- Examples
- #1 – Continuous Compounding
- #2 – Daily Compounding
- #3 – Monthly Compounding
- #4 – Quarterly Compounding
- #5 – Half-yearly Compounding
- #6 – Annual Compounding
- Relevance and Use
- Recommended Articles
Steps to Calculate Effective Annual Rate (EAR)
- Firstly, figure out the nominal rate of interest for the given investment, and it is easily available at the stated rate of interest. The nominal rate of interest is denoted by 'r.'
- Next, try to determine the number of compounding periods per year, and the compounding can be quarterly, half-yearly, annually, etc. The number of compounding periods of nominal interest rate per year is denoted by 'n.' (The step is not required for continuous compounding)
- Finally, in the case of discrete compounding, the calculation of Effective Annual Rate can be done using the following equation as,
Effective Annual Rate = (1 + r/n)n – 1
On the other hand, in case of continuous compounding, the calculation of Effective Annual Rate can be done using the following equation as,
Effective Annual Rate = er – 1
Examples
You can download this Effective Annual Rate Formula Excel Template here – Effective Annual Rate Formula Excel Template
Let us take an example where the effective annual rate is to be calculated for one year with the nominal or stated rate of interest of 10%. Calculate the effective annual rate for the following compounding period:
- Continuous
- Daily
- Monthly
- Quarterly
- Half Yearly
- Annual
Given, Nominal rate of interest, r = 10%
#1 – Continuous Compounding
The calculation of EAR is done using the above formula as,
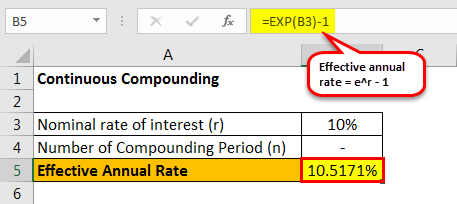
Effective annual rate = er – 1
Effective annual rate = e12% – 1 = 10.5171%
#2 – Daily Compounding
Since daily compounding, therefore n = 365
The calculation of Effective Annual Rate is done using the above formula as,

Effective annual rate = (1 + r/n)n – 1
Effective annual rate = (1 + 10%/365) 365 – 1 = 10.5156%
#3 – Monthly Compounding
Since monthly compounding, therefore n = 12
The calculation of Effective Annual Rate is done using the above formula as,
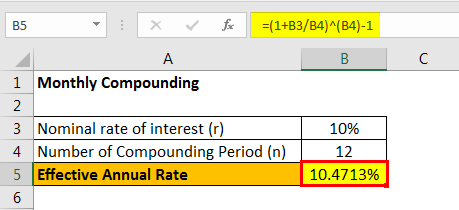
Effective annual rate = (1 + 10%/12)12 – 1 = 10.4713%
#4 – Quarterly Compounding
Since quarterly compounding The compounding quarterly formula depicts the total interest an investor can earn on investment or financial product if the interest is payable quarterly and reinvested in the scheme. It considers the principal amount, quarterly compounded rate of interest and the number of periods for computation. read more , therefore n = 4
The calculation of EAR is done using the above formula as,

Effective annual rate = (1 + 10%/4)4 – 1 = 10.3813%
#5 – Half-yearly Compounding
Since half yearly compounding, therefore n = 2
The calculation of Effective Annual Rate is done using the above formula as,

Effective annual rate = (1 + 10%/2)2 – 1 = 10.2500%
#6 – Annual Compounding
Since annual compounding, therefore n = 1
The calculation of Effective Annual Rate is done using the above formula as,

Effective annual rate = (1 + 10%/1)1 – 1 = 10.0000%
The above example shows that the formula for EAR depends not only on the nominal or stated rate of interest of the investment but also on how many times the rate compounding happens during a year, and it increases with the increase in the number of compounding per year.

The below-given graph shows the rate of compounding happens during a year

Relevance and Use
The concept of an effective annual rate is an indispensable part of investing for a financial user since it is the interest rate effectively received from an investment. Further, an investor will be benefited in case the effective interest rate is higher than the nominal rate of interest offered by the issuer.
From a borrower's point of view, also it is vital to understand the concept of an effective annual rate because it will impact their solvency Solvency of a company means its ability to meet the long term financial commitments, continue its operation in the foreseeable future and achieve long term growth. It indicates that the entity will conduct its business with ease. read more and profitability. A higher expense towards interest payment eventually lowers the interest coverage ratio The interest coverage ratio indicates how many times a company's current earnings before interest and taxes can be used to pay interest on its outstanding debt. It can be used to determine a company's liquidity position by evaluating how easily it can pay interest on its outstanding debt. read more for a borrower that could negatively impact the borrower's ability to service the debt in the future. Further, a higher interest expense Interest expense is the amount of interest payable on any borrowings, such as loans, bonds, or other lines of credit, and the costs associated with it are shown on the income statement as interest expense. read more also reduces the net income and profitability of a company (all other factors being equal).
The effective interest rate is one of the simplest forms of interest rate, and in the actual monetary terms, it is basically the rate at which a borrower pays to a lender to use its money. Further, the concept of effective annual rate also encapsulates the impact of no. of compounding per year, which eventually helps in the calculation of redemption value at maturity. Normally, the effective annual rate is greater than the nominal rate of interest because the nominal rate is expressed in terms of yearly percentage irrespective of the number of compounding per year.
If we increase the number of compounding periods, then the effective annual rate also increases in line with the nominal rate. Additionally, if an investment is compounded annually, then it will have an effective annual rate, which is exactly equal to the nominal rate of interest. On the other hand, if the investor had invested on a quarterly compounding basis, then the effective annual rate would be greater than the nominal rate of interest.
Recommended Articles
This article has been a guide to Effective Annual Rate Formula. Here we discuss how to calculate Effective Annual Rate (EAR) along with the practical example and downloadable excel sheet. You can learn more about accounting from the following articles –
- Formula of Real Interest Rate The Real Interest Rate formula calculates the rate of interest after excluding the impact of inflation. It helps measure inflation-adjusted return on investments on financial security or a loan or deposits. Real interest rate formula = normal rate of interest - inflation (actual or expected) read more
- Nominal vs. Real Interest Rate The nominal rate is that rate of return which considers the monetary value but ignores inflation during calculation. In contrast, the real rate includes inflation for computation and thus provides transparency to the investors by reflecting the opportunity value. read more
- Nominal Interest Rate Nominal Interest rate refers to the interest rate without the adjustment of inflation. It is a short term interest rate which is used by the central banks to issue loans. read more
Source: https://www.wallstreetmojo.com/effective-annual-rate-formula/
0 Response to "How to Convert Effective Rate to Continuous Rate"
Post a Comment